Classical and Quantum Strategies to Boost Quantum Subspace Methods
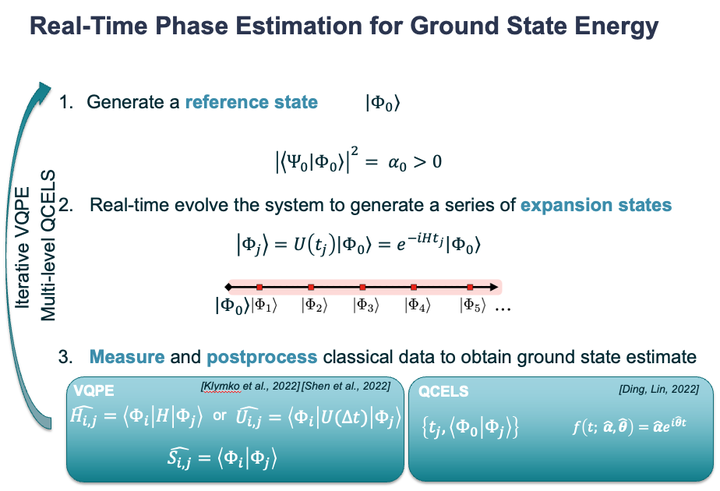 QSM based on real-time dynamics.
QSM based on real-time dynamics.
Abstract
Quantum subspace methods are an exciting class of hybrid quantum algorithms for ground and excited state computations where approximate energies are extracted from an appropriate subspace of the full Hilbert space. The expansion states that form the basis for the subspace are prepared on the quantum computer and the projected problem is retrieved through measurement. The approximate energies are then obtained through classical diagonalization of the low-dimensional projected problem. In this talk, we present classical and quantum strategies that aim to improve the energy approximations and convergence behavior of quantum subspace algorithms by improving the conditioning of the basis of expansion states through both implicit and explicit methods. We show that our strategies lead to more accurate energies for comparable classical and quantum resources and illustrate the performance through numerical simulations for a variety of problems stemming from condensed matter physics and electronic structure theory.