Measurement cost of a two-dimensional Fermi-Hubbard Hamiltonian.
Abstract
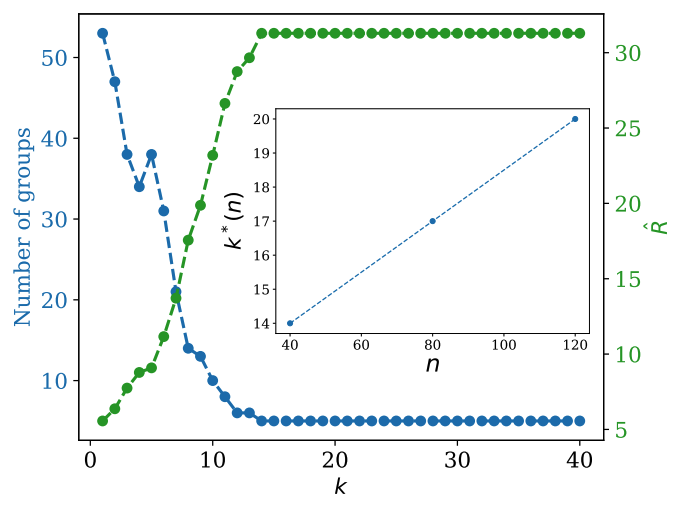
We introduce a notion of commutativity between operators on a tensor product space, nominally Pauli strings on qubits, that interpolates between qubit-wise commutativity and (full) commutativity. We apply this notion, which we call k-commutativity, to measuring expectation values of observables in quantum circuits and show a reduction in the number measurements at the cost of increased circuit depth. Last, we discuss the asymptotic measurement complexity of k-commutativity for several families of n-qubit Hamiltonians, showing examples with O(1), O(√n), and O(n) scaling.
Daan Camps
Researcher in Advanced Technologies Group
My research interests include quantum algorithms, numerical linear algebra, tensor factorization methods and machine learning. I’m particularly interested in studying the interface between HPC and quantum computing.